By Department of Mathematics
Deadline: 11:59 p.m. on Sunday, Sept. 8, 2019
The Rules:
The contest is open to everyone. Individuals or teams of at most three members may submit solutions. Do not discuss the problem with anyone other than members of your team. You may use any source, written or electronic, but all sources must be properly cited. You may use any computational tools. Your solution will be graded on a 0–4 point scale. All your assertions must be completely and fully justified. At the same time, you should aim to be as concise as possible; avoid overly lengthy arguments and unnecessary components. Your grade will be based on both mathematical accuracy and clarity of presentation. Either send your solution to bbajnok@gettysburg.edu or put it in Bela’s departmental mailbox by the above deadline. Consistently successful participants will receive the Paul Mugabi Mathematics Problem Solving Award.
The Problem:
Suppose that each of the quadrilaterals ACHF, ABHG, and AEHD in the figure below are parallelograms. (The figure is not drawn to scale.)
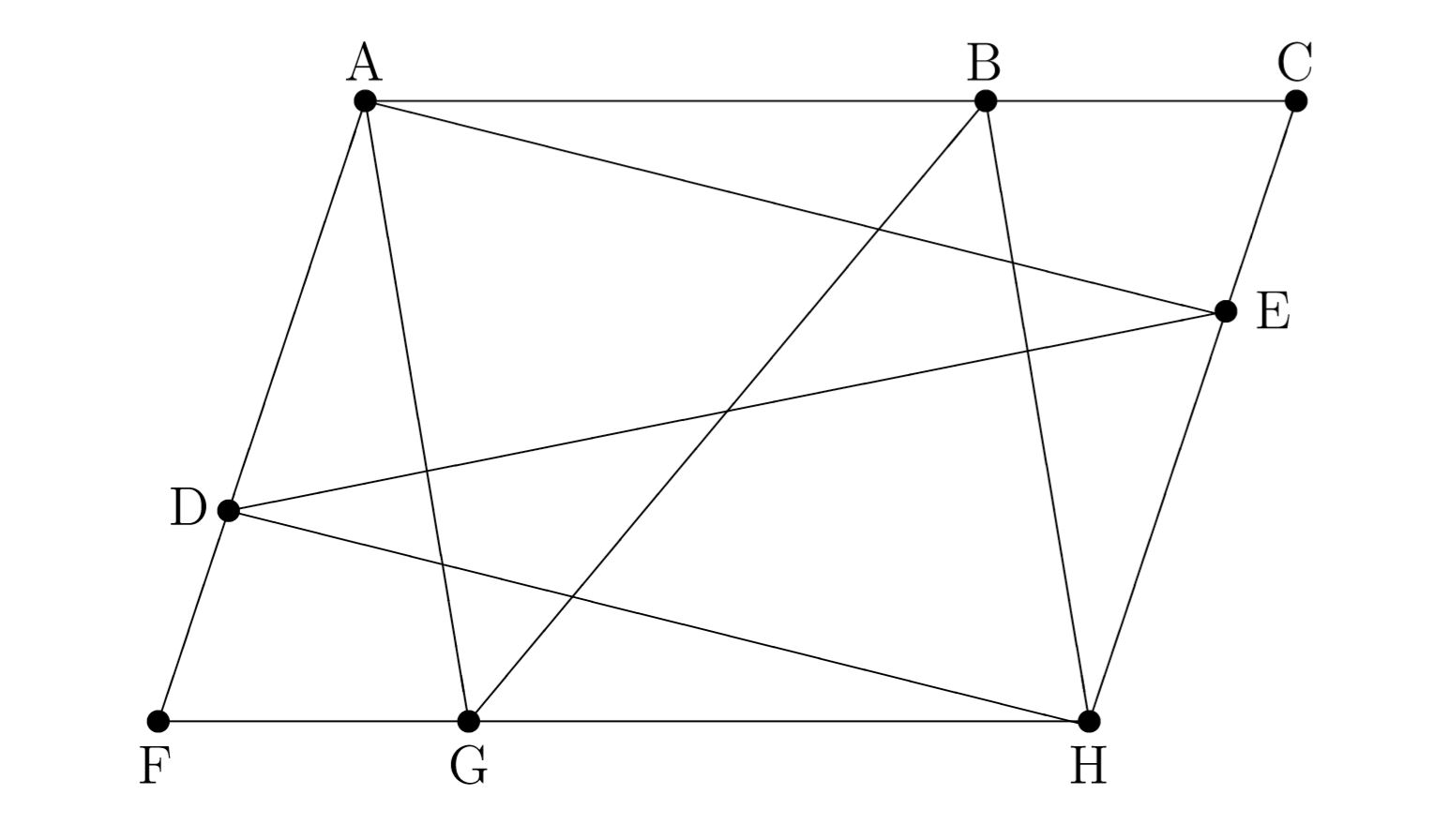
Color each of the fourteen regions by either orange or blue in such a way that regions sharing a boundary line receive different colors. There are exactly two ways to do this; consider the one that has six orange regions and eight blue regions (the other coloring will be the reverse). Prove that it is possible to partition the six orange regions into two groups so that each group contains three regions, and the sum of the areas of the three regions in one group equals the sum of the areas of the three regions in the other group.
