Editor’s Note: The Department of Mathematics at Gettysburg College hosts a problem of the week challenge to determine each semester’s Paul Mugabi problem-solving award recipient(s). Each week’s entries are scored by a faculty judge, and winner(s) from each week will receive a Problem Of the Week (P.O.W.) button. The Gettysburgian is not involved in or responsible for accepting or evaluating students’ submissions to this contest.
Solutions Due: Friday, October 6, 5:00 p.m.
Send solutions to bkennedy@gettysburg.edu.
The contest is open to all Gettysburg College students. Up to three people may work together on a submission. Make sure your name is on your submission and that any sources are properly cited. Weekly winners will receive a POW victory button, and the best-performing students of the semester will receive the Paul Mugabi problem-solving award.
Let’s Gamble!
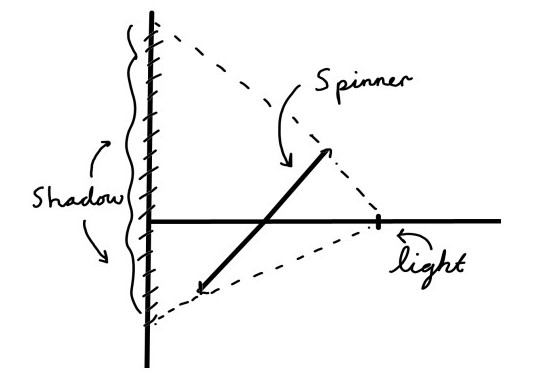
There is a spinner of radius 1 (so of total length 2) with its center at point (1, 0) in the plane. A light source is at (2, 0). We will spin the spinner and, once it stops, shine the light source and look at the length of the shadow of the spinner on the y-axis. See the figure below.
Problem of the Week: Fall 2023, Number 6
Consider the following game: you pay a dollar, and then we spin the spinner and turn on the light. If the length of the shadow is greater than 8, you get back your dollar plus another dollar.
If the length of the shadow is less than 8, you lose your dollar. Would you play this game? Explain.
