Problem of the Week: Fencing Lessons
Editor’s Note: The Department of Mathematics at Gettysburg College hosts a problem of the week challenge to determine each semester’s Paul Mugabi problem-solving award recipient(s). Each week’s entries are scored by a faculty judge, and winner(s) from each week will receive a Problem Of the Week (P.O.W.) button. The Gettysburgian is not involved in or responsible for accepting or evaluating students’ submissions to this contest.
THE RULES:
The contest is open to all Gettysburg College students. Up to three people may work together on a submission. Make sure your name is on your submission and that any sources are properly cited. Send solutions to bkennedy@gettysburg.edu. This problem was posted on Friday, February 4 and solutions are due on Friday, February 11 by 5:00 p.m.
THE PROBLEM:
We have exactly 20 yards of fencing; we are going to use this fencing to enclose a little garden. We have decided on the following rules:
- all the sides of the garden must point either exactly north-south or exactly east-west;
- all the sides of the garden must be a whole number of yards long;
- we must use all the fencing, and we must be able to walk along all 20 yards of fencing either inside or outside the garden (so the fencing can’t “fold flat” anywhere, or “pinch together” to create two essentially separate gardens).
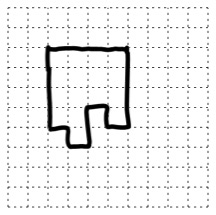
The picture below shows an allowed garden.
(Figure courtesy of Professor Benjamin Kennedy)
THE QUESTION:
What areas of the garden are possible? Answer, and explain.
